5.2 - Evolution of Integration
Riemann sums
- A way to approximate (and eventually obtain) an integral
- Using techniques like LRAM, MRAM, RRAM, and the Trapezoidal Rule, we can estimate integrals
- As we create more and more partitions, we can estimate integrals even better
- Eventually, we will be drawing infinitesimally small integrals and getting an exact area
- Taking a Riemann sum involves drawing rectangles under a curve
- Eventually, we take the limit of these rectangles as the number of them () approaches infinity and find their combined area.
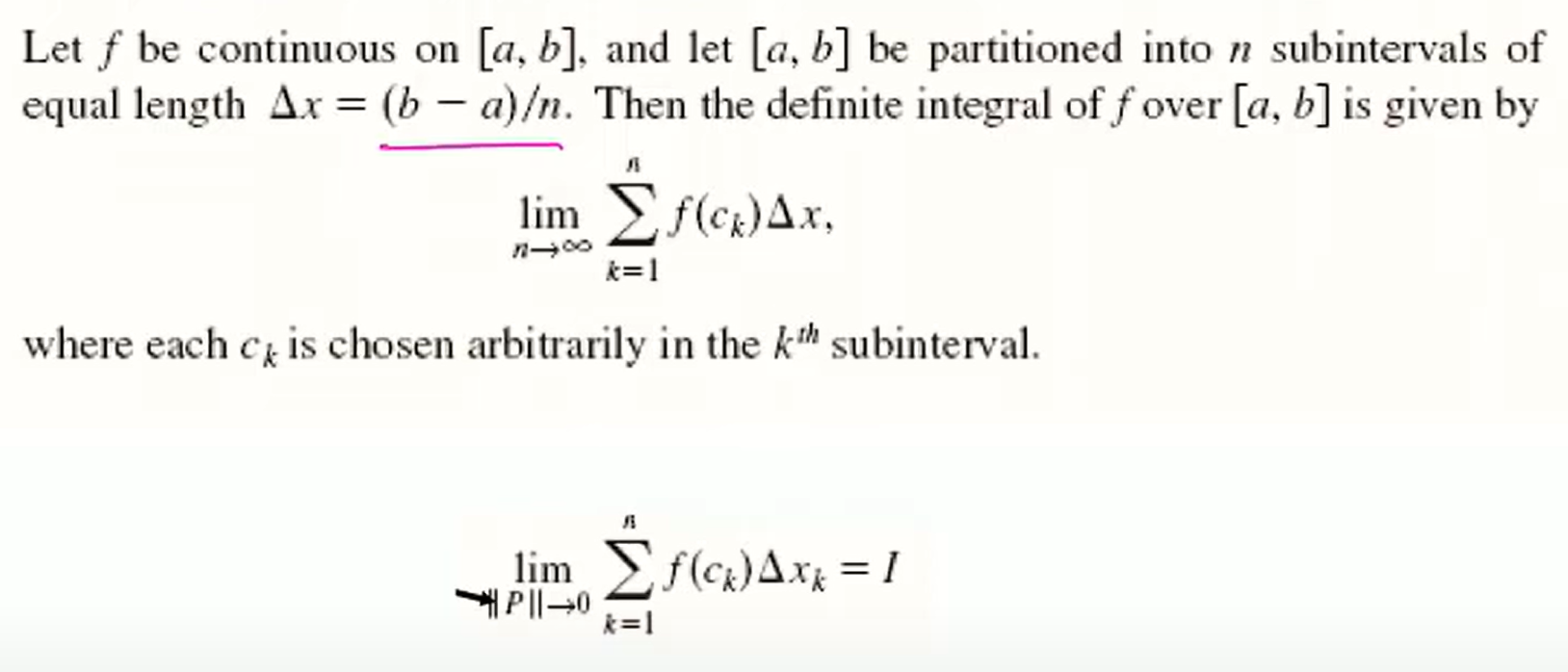
- Notice that the is the length of each equal partition.
- Some problems might involve going from this to integral notation: . Substitute for to convert between forms.
Some facts
- All continuous functions are integrable (able to be integrated).
